TESSERACT
Shadow of a higher dimension
New: TSSRKT is available on OBJKT Hic et nunc [4/4]
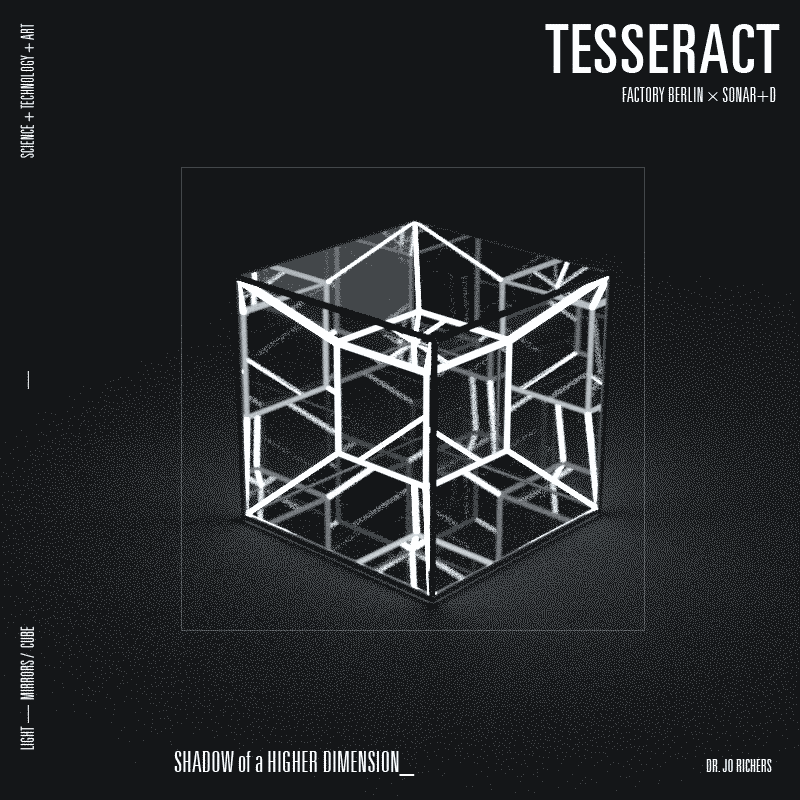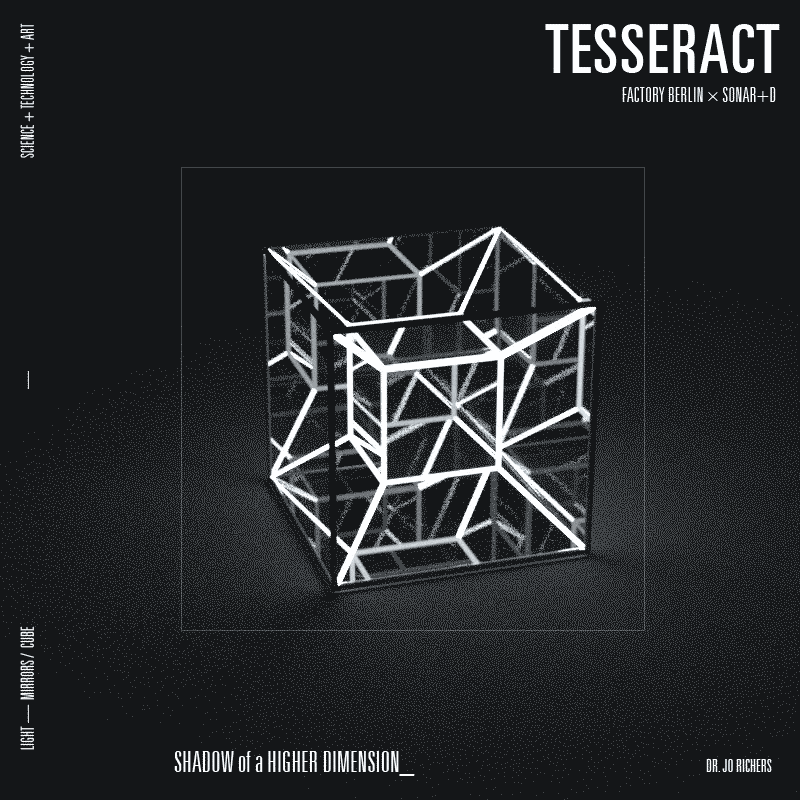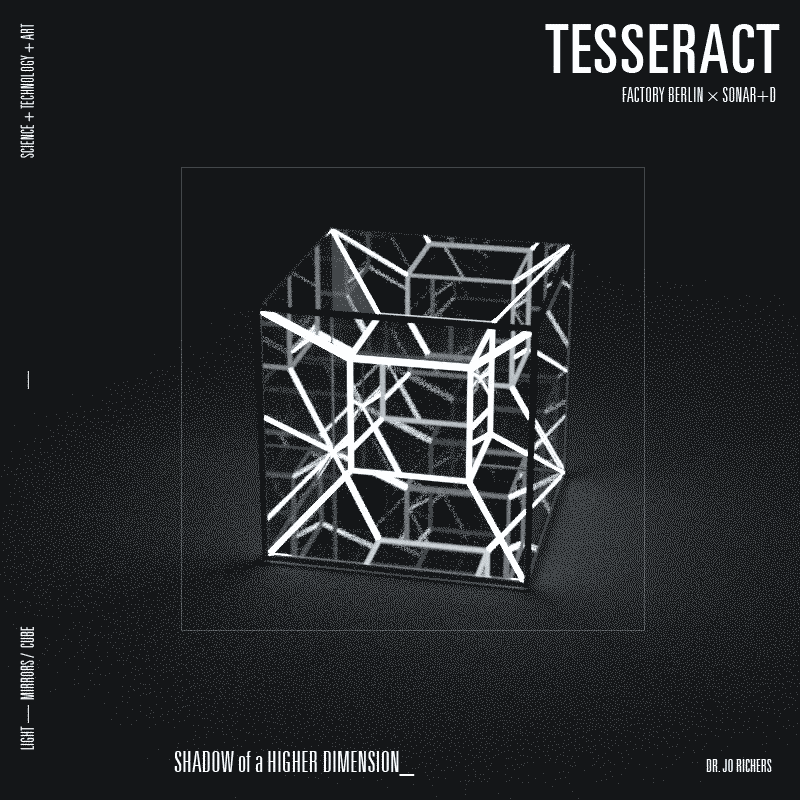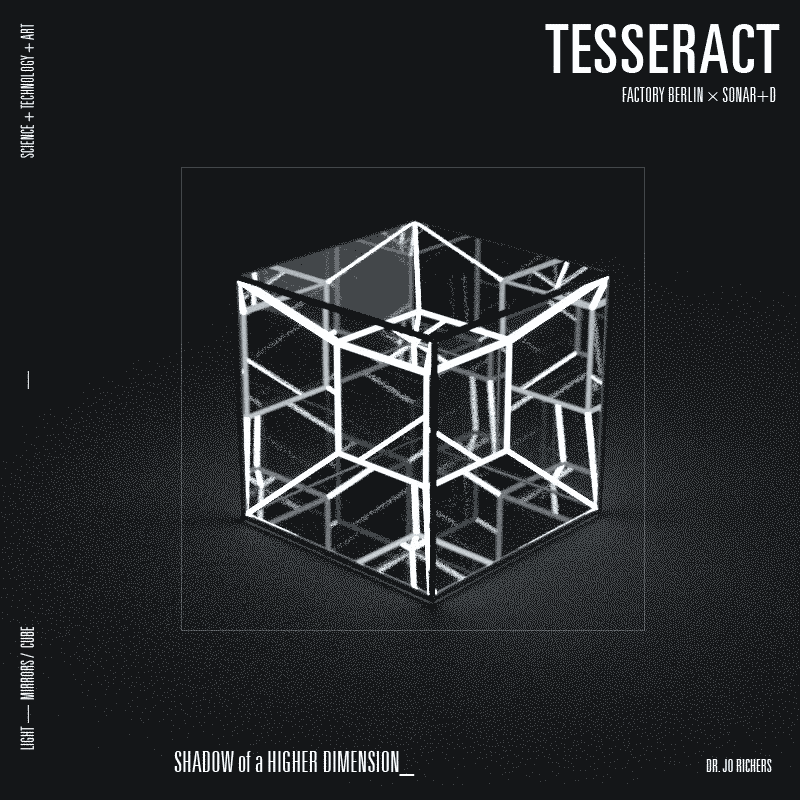
Figure 01: Animated 3D-rendering of the infinity mirrored four-dimensional cube (Cinema4d/Octane)
Although our world is bound to three dimensions, higher-dimensional objects can be constructed not only as abstract mathematical concepts. For example, in geometry, the tesseract is the higher-dimensional analogue of the cube.[1] It is described by a hypersurface that consists of eight cubical cells. Hence, a model can be designed that represents all eight cubes in one arrangement following the proportions of the silver ratio.
Figure 02: Frames of the first stereoscopic 3D computer animation of a tesseract by A. Michal Noll
»The commonality between science and art is in trying to see profoundly – to develop strategies of seeing and showing.« (Edward R. TUFTE).
Light, mirrors and the »shadow« of a hypercube: TESSERACT explores the theme of multi-dimensionality and offers new insights into the representational possibilities emerging from the intersection of science, technology and art. At the same time, it serves as a reflection of the interdisciplinary praxis:[2] The concept of the tesseract itself, deeply rooted in scientific thinking and mathematical abstraction, is manifested through technology by leveraging prototyping, construction and programming.[3] Passed through the lens of design with a focus on human perception and proportion principles, a communicative purpose is defined—a strategy of showing. Similar to the mirrors that reflect a seemingly endless array of light, finally, art as the primary mode is expressed through the sculptural object allowing the viewer to see profoundly. Just as the tesseract displays what happens to the cubic form when a fourth dimension is added, it serves as an allegory of the innovation space defined by interdisciplinary praxis and becomes a meta image for its depth and potential.
Figure 3: Krebs Cycle of creativity by Neri Oxman
Figure 03: Internal proportions and aspect ratios of the Eight-cell (side view only)
Figure 04: Geometric construction of the silver ratio tesseract (2D projection)
Prototype with 3D-printed modular corner pieces (in collaboration with Caroline Barrueco)
Figure 05: Photograph of the black tesseract (Wood, PLA-filament print, black acryl)
Figure 06: 3d-printed corner piece and connection node
About:
Dr. Jo Richers is a scientist and designer. He is a guest lecturer at the Technical University of Berlin, artist in residence with Factory Berlin × Sonar+D and runs a studio for visual science communication. He explores the intersection of science, art, and design, either through personal projects or in collaboration with international partners and clients from academic and industrial research.
References
[1] A. Michael Noll, “Hypercube (1965) - First stereoscopic 3D computer animation”, YouTube, https://youtu.be/RqQvVts5Yj0, accessed January 2020
[2] Neri Oxman, “Age of entanglement,” Journal of Design and Science, January 14 2016, https://jods.mitpress.mit.edu/pub/ageofentanglement, accessed December 2019
[3] Henry Segerman, Visualizing Mathematics with 3D Printing, 2016, Johns Hopkins University Press